(Solved): A right circular cylinder is placed inside a cone of radius \( \mathrm{R} \) and height \( \mathrm ...

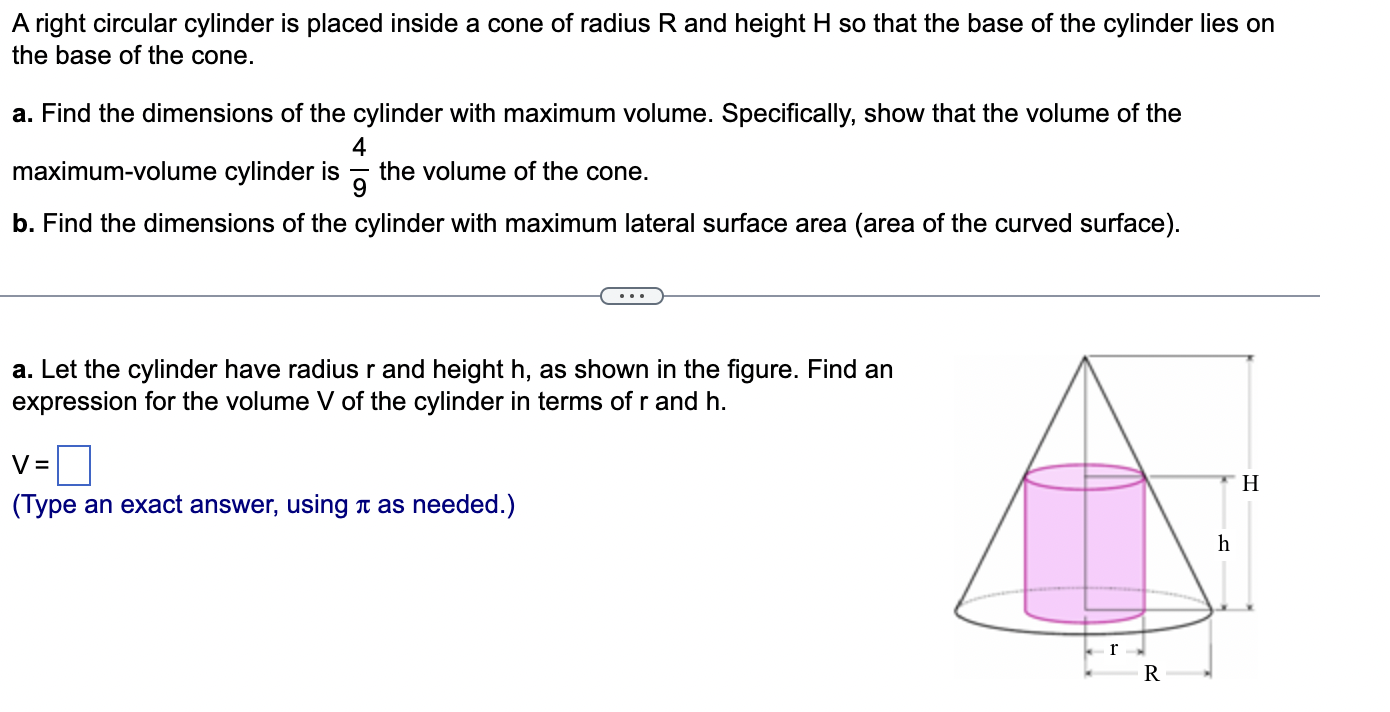
A right circular cylinder is placed inside a cone of radius \( \mathrm{R} \) and height \( \mathrm{H} \) so that the base of the cylinder lies on the base of the cone. a. Find the dimensions of the cylinder with maximum volume. Specifically, show that the volume of the maximum-volume cylinder is \( \frac{4}{9} \) the volume of the cone. b. Find the dimensions of the cylinder with maximum lateral surface area (area of the curved surface). a. Let the cylinder have radius \( r \) and height \( h \), as shown in the figure. Find an expression for the volume \( V \) of the cylinder in terms of \( r \) and \( h \). \[ \mathrm{V}= \] (Type an exact answer, using \( \pi \) as needed.)