Home /
Expert Answers /
Advanced Math /
a-consider-the-finite-field-z-3-x-x-3-x-2-x-2-let-alpha-x-2-x-evaluate-all-powers-pa947
(Solved): a) Consider the finite field Z_(3)[x] (x^(3)+x^(2)+x+2:). Let \alpha =x^(2)+x. Evaluate all powers ...
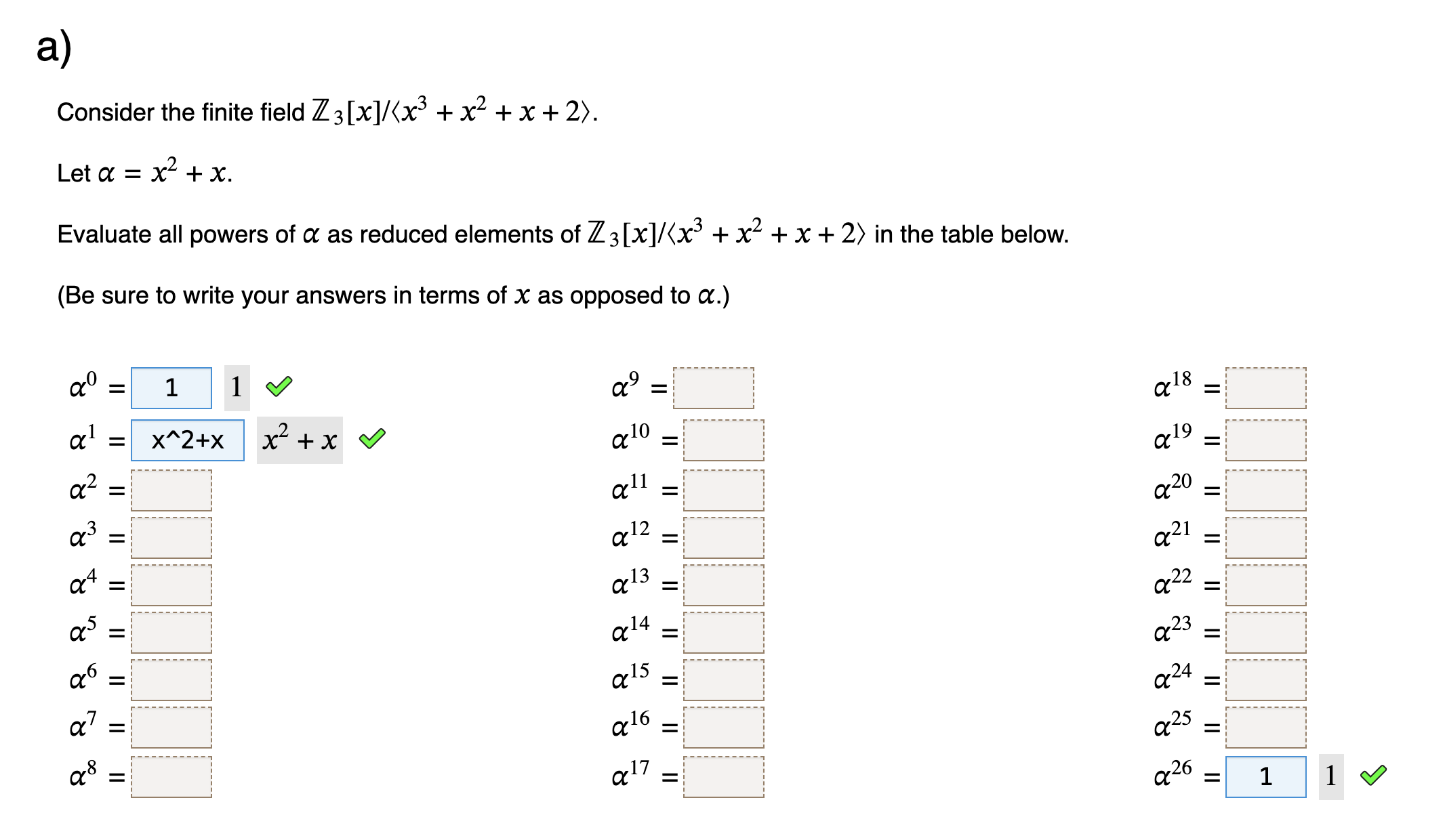
a) Consider the finite field
Z_(3)[x] (x^(3)+x^(2)+x+2:). Let
\alpha =x^(2)+x. Evaluate all powers of
\alpha as reduced elements of Z_(3)[x] (x^(3)+x^(2)+x+2).in the table below. (Be sure to write your answers in terms of
xas opposed to
\alpha .)
\alpha ^(0)=,1
\alpha ^(1)=◻
\alpha ^(2)=◻
\alpha ^(3)=◻
\alpha ^(4)=◻
\alpha ^(5)=
\alpha ^(6)=
\alpha ^(7)=
\alpha ^(8)=
\alpha ^(9)=,\alpha ^(18)=
\alpha ^(10)=,\alpha ^(19)=
\alpha ^(11)=,\alpha ^(20)=
\alpha ^(12)=,\alpha ^(21)=
\alpha ^(13)=,\alpha ^(22)=
\alpha ^(14)=,\alpha ^(23)=
\alpha ^(15)=,\alpha ^(24)=
\alpha ^(16)=,\alpha ^(25)=
\alpha ^(17)=,\alpha ^(26)=b)Hence write (2x^(2)+2)/(x+2) as a reduced element of Z_(3)[x] (x^(3)+x^(2)+x+:).