(Solved): 5. Using the \( \epsilon-N \) argument to show that the following sequences \( \left\{a_{n}\right\ ...

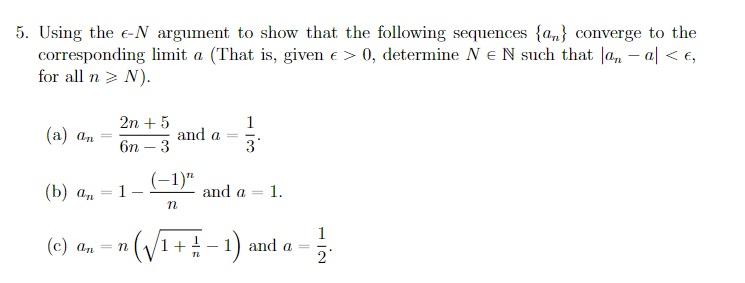
5. Using the \( \epsilon-N \) argument to show that the following sequences \( \left\{a_{n}\right\} \) converge to the corresponding limit \( a \) (That is, given \( \epsilon>0 \), determine \( N \in \mathbb{N} \) such that \( \left|a_{n}-a\right|<\epsilon \), for all \( n \geqslant N) \). (a) \( a_{n}=\frac{2 n+5}{6 n-3} \) and \( a=\frac{1}{3} \). (b) \( a_{n}=1-\frac{(-1)^{n}}{n} \) and \( a=1 \). (c) \( a_{n}=n\left(\sqrt{1+\frac{1}{n}}-1\right) \) and \( a=\frac{1}{2} \).