Home /
Expert Answers /
Advanced Math /
21-in-a-group-g-let-c-be-the-center-and-c-a-be-the-centralizer-of-a-pr-pa148
(Solved): 21. In a group \( G \), let \( C \) be the center and \( C_{a} \) be the centralizer of \( a \). Pr ...
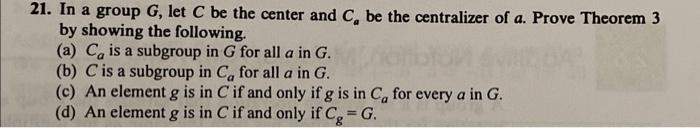

21. In a group \( G \), let \( C \) be the center and \( C_{a} \) be the centralizer of \( a \). Prove Theorem 3 by showing the following. (a) \( C_{a} \) is a subgroup in \( G \) for all \( a \) in \( G \). (b) \( C \) is a subgroup in \( C_{a} \) for all \( a \) in \( G \). (c) An element \( g \) is in \( C \) if and only if \( g \) is in \( C_{a} \) for every \( a \) in \( G \). (d) An element \( g \) is in \( C \) if and only if \( C_{g}=G \).
3 Centralizers and the Center In a group \( G \), let \( C \) be the center and \( C_{a} \) be the centralizer of \( a \). Then: (a) \( C_{a} \) is a subgroup in \( G \) for every \( a \) in \( G \). (b) \( C \) is a subgroup in \( C_{a} \) for every \( a \) in \( G \). (c) An element \( g \) is in \( C \) if and only if \( g \) is in \( C_{a} \) for every \( a \) in \( G \). (d) An element \( g \) is in \( C \) if and only if \( C_{g}=G \). The proof of this result is left to the reader as Problem 21 of this section.
Expert Answer
(3). Let G be a group. Center of a Group: The center of a group G is the set of elements that commute with every element of G, denoted by C. C={g?G?ga