(Solved): 2. The force exerted by an electric charge at the origin on a charged particle at a point \( (x, y ...

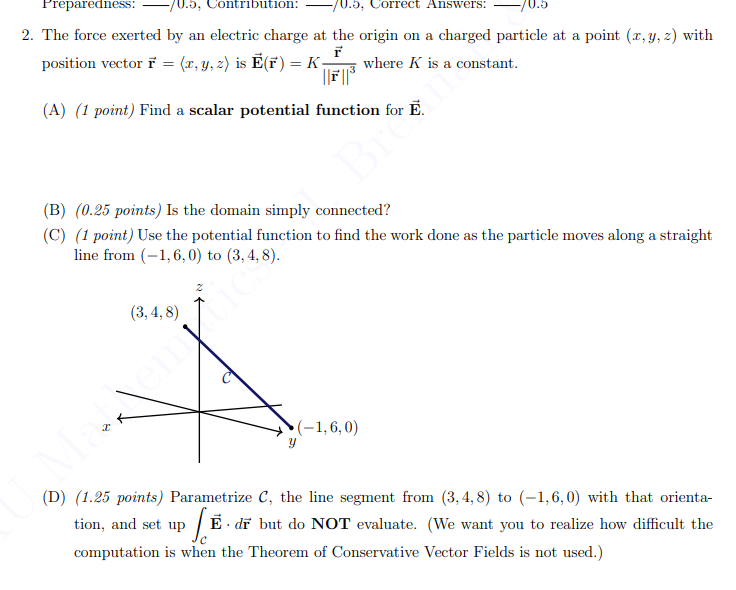
2. The force exerted by an electric charge at the origin on a charged particle at a point \( (x, y, z) \) with position vector \( \overrightarrow{\mathbf{r}}=\langle x, y, z\rangle \) is \( \overrightarrow{\mathbf{E}}(\overrightarrow{\mathbf{r}})=K \frac{\overrightarrow{\mathbf{r}}}{\|\overrightarrow{\mathbf{r}}\|^{3}} \) where \( K \) is a constant. (A) (1 point) Find a scalar potential function for \( \overrightarrow{\mathbf{E}} \). (B) (0.25 points) Is the domain simply connected? (C) (1 point) Use the potential function to find the work done as the particle moves along a straight line from \( (-1,6,0) \) to \( (3,4,8) \). (D) (1.25 points) Parametrize \( \mathcal{C} \), the line segment from \( (3,4,8) \) to \( (-1,6,0) \) with that orientation, and set up \( \int_{\mathcal{C}} \overrightarrow{\mathbf{E}} \cdot d \overrightarrow{\mathbf{r}} \) but do NOT evaluate. (We want you to realize how difficult the computation is when the Theorem of Conservative Vector Fields is not used.)