(Solved): 2. A thin spherical shell of charge with radius \( R \) is centered at the origin and is immerse ...

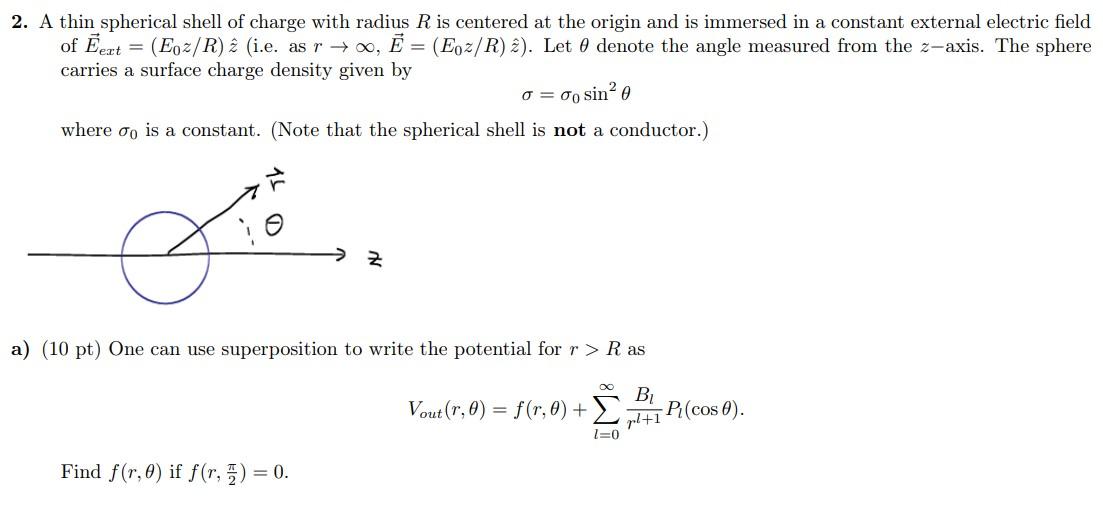
2. A thin spherical shell of charge with radius \( R \) is centered at the origin and is immersed in a constant external electric field of \( \vec{E}_{e x t}=\left(E_{0} z / R\right) \hat{z} \) (i.e. as \( \left.r \rightarrow \infty, \vec{E}=\left(E_{0} z / R\right) \hat{z}\right) \). Let \( \theta \) denote the angle measured from the \( z \)-axis. The sphere carries a surface charge density given by \[ \sigma=\sigma_{0} \sin ^{2} \theta \] where \( \sigma_{0} \) is a constant. (Note that the spherical shell is not a conductor.) a) (10 pt) One can use superposition to write the potential for \( r>R \) as \[ V_{\text {out }}(r, \theta)=f(r, \theta)+\sum_{l=0}^{\infty} \frac{B_{l}}{r^{l+1}} P_{l}(\cos \theta) . \] Find \( f(r, \theta) \) if \( f\left(r, \frac{\pi}{2}\right)=0 \). (20pt) Suppose \( E_{0}=0 \). What is the value of the potential at \( r=0 \) ? [hint: Note only \( l=0 \) mode contributes to \( r=0 \).]