Home /
Expert Answers /
Advanced Math /
1-why-do-we-need-one-initial-condition-for-a-first-order-differential-equation-two-initial-conditi-pa339
(Solved): 1) Why do we need one initial condition for a first order differential equation, two initial conditi ...
1) Why do we need one initial condition for a first order differential equation, two initial conditions for a second
order differential equation, three initial conditions for a third order differential equation?
2) Green's functions also exist for certain periodic Sturm-Liouville problems. Explain how to construct a Green's
function for the problem. Do not solve for finding y.
y^('')+2y=-x,y(0)=y(2\pi ),y^(')(0)=y^(')(2\pi )
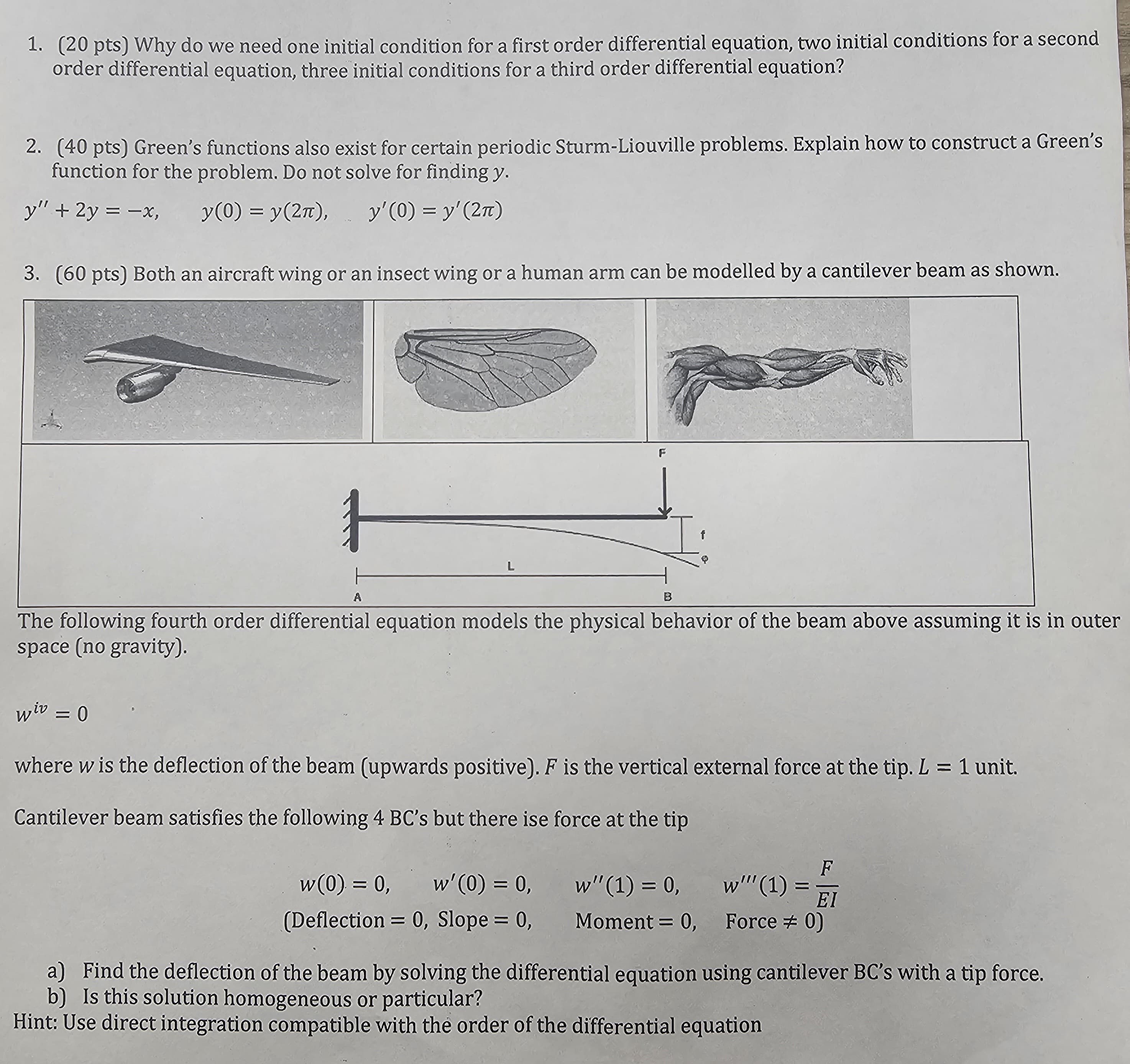
3) Both an aircraft wing or an insect wing or a human arm can be modelled by a cantilever beam as shown.
The following fourth order differential equation models the physical behavior of the beam above assuming it is in outer
space (no gravity).
w^(iv)=0
where w is the deflection of the beam (upwards positive). F is the vertical external force at the tip. L=1 unit.
Cantilever beam satisfies the following 4 BC's but there ise force at the tip
w(0)=0,w^(')(0)=0,w^('')(1)=0,
w^(''')(1)=(F)/(EI)
(Deflection =0, Slope =0, Moment =0, Force !=0)
a) Find the deflection of the beam by solving the differential equation using cantilever BC's with a tip force.
b) Is this solution homogeneous or particular?