(Solved): 1. The Magnus effect is the observation that a rotating sphere in flight experiences an aerodynamic ...

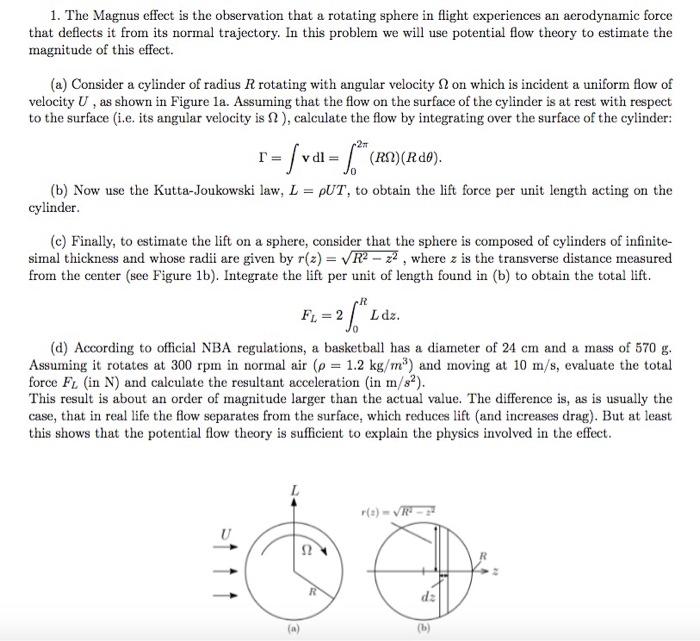
1. The Magnus effect is the observation that a rotating sphere in flight experiences an aerodynamic force that deflects it from its normal trajectory. In this problem we will use potential flow theory to estimate the magnitude of this effect. (a) Consider a cylinder of radius \( R \) rotating with angular velocity \( \Omega \) on which is incident a uniform flow of velocity \( U \), as shown in Figure 1a. Assuming that the flow on the surface of the cylinder is at rest with respect to the surface (i.e. its angular velocity is \( \Omega \) ), calculate the flow by integrating over the surface of the cylinder: \[ \Gamma=\int \mathrm{v} \mathrm{dl}=\int_{0}^{2 \pi}(R \Omega)(R \mathrm{~d} \theta) . \] (b) Now use the Kutta-Joukowski law, \( L=\rho U T \), to obtain the lift force per unit length acting on the cylinder. (c) Finally, to estimate the lift on a sphere, consider that the sphere is composed of cylinders of infinitesimal thickness and whose radii are given by \( r(z)=\sqrt{R^{2}-z^{2}} \), where \( z \) is the transverse distance measured from the center (see Figure 1b). Integrate the lift per unit of length found in (b) to obtain the total lift. \[ F_{L}=2 \int_{0}^{R} L \mathrm{~d} z . \] (d) According to official NBA regulations, a basketball has a diameter of \( 24 \mathrm{~cm} \) and a mass of \( 570 \mathrm{~g} \). Assuming it rotates at \( 300 \mathrm{rpm} \) in normal air \( \left(\rho=1.2 \mathrm{~kg} / \mathrm{m}^{3}\right) \) and moving at \( 10 \mathrm{~m} / \mathrm{s} \), evaluate the total force \( F_{L} \) (in \( \mathrm{N} \) ) and calculate the resultant acceleration (in \( \mathrm{m} / \mathrm{s}^{2} \) ). This result is about an order of magnitude larger than the actual value. The difference is, as is usually the case, that in real life the flow separates from the surface, which reduces lift (and increases drag). But at least this shows that the potential flow theory is sufficient to explain the physics involved in the effect.