(Solved): 1. Set up the differential amplifier circuit shown below and adjust the supply voltage to \( + ...

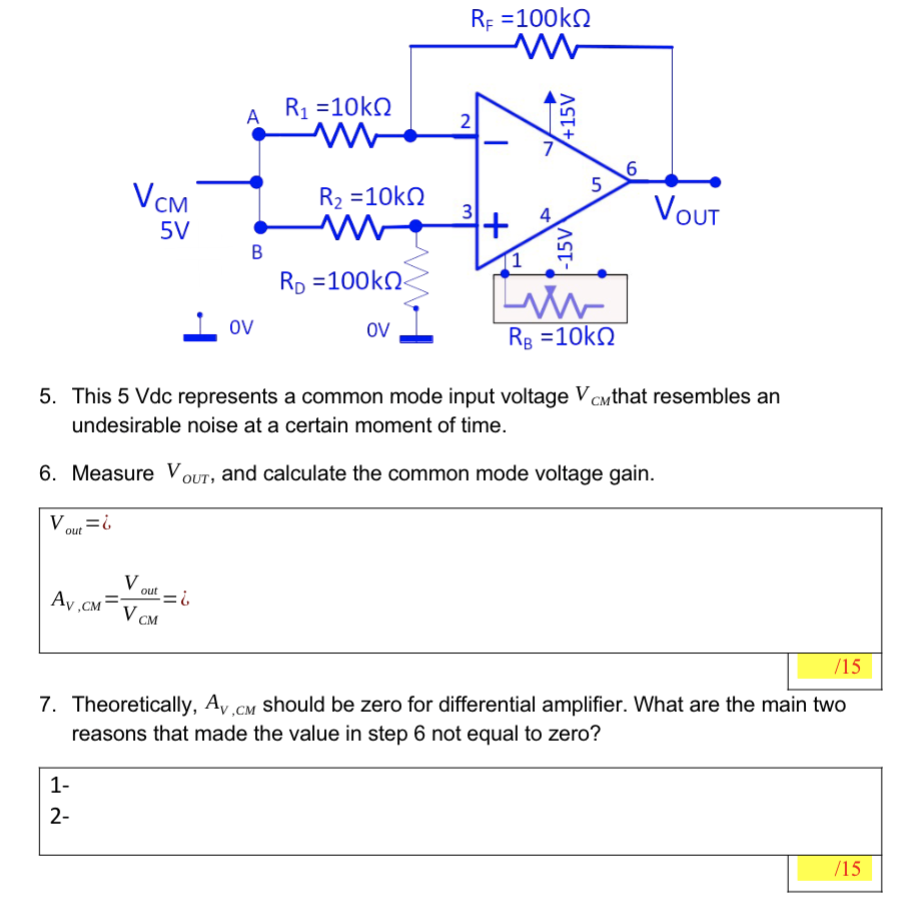
1. Set up the differential amplifier circuit shown below and adjust the supply voltage to \( +/-15 V D C \). 2. This circuit is a differential amplifier circuit with additional potentiometer \( R_{\mathbf{B}} \) to null the output-offset voltage. Make a short circuit between nodes A, B and the common 0 , then adjust \( R_{B} \) until \( V_{\text {out }} \) is equal to zero millivolts. B- Calculating the differential voltage gain \( A_{v} \) 3. The differential voltage gain can be measured or calculated using the equation \( A_{V}=\frac{-R_{F}}{R_{1}} \). Calculate the theoretical differential-mode voltage gain \( A_{V} \). \[ A_{V}=\frac{-R_{F}}{R_{1}}=i \] 5. This \( 5 \mathrm{Vdc} \) represents a common mode input voltage \( V_{C M} \) that resembles an undesirable noise at a certain moment of time. 6. Measure \( V_{\text {out }} \), and calculate the common mode voltage gain. 7. Theoretically, \( A_{V, C M} \) should be zero for differential amplifier. What are the main two reasons that made the value in step 6 not equal to zero? 10. The highest CMRR occurs as a result of having matched resisters \( \left(R_{2}=R_{1}\right. \) and \( R_{D}=R_{F} \) ). To prove this, make a mismatch between \( R_{D} \) and \( R_{F} \) by making \( R_{D}=82 k \Omega \) while keeping \( R_{F}=100 \mathrm{k} \Omega \). Measure \( V_{\text {out }} \) then calculate the new \( A_{v . c m} \) and CMRR. (note \( A_{V} \) will not change in this case) \[ \begin{array}{l} V_{\text {out }}=i \\ A_{V, C M}=\frac{V_{\text {out }}}{V_{C M}}=i \end{array} \] \[ C M R R=20 \log _{10}\left|\frac{A_{V}}{A_{V, C M}}\right|=\zeta \]