(Solved): (1 point) Let \( f: \mathbb{R}^{2} \rightarrow \mathbb{R}^{2} \) be the linear transformation defin ...

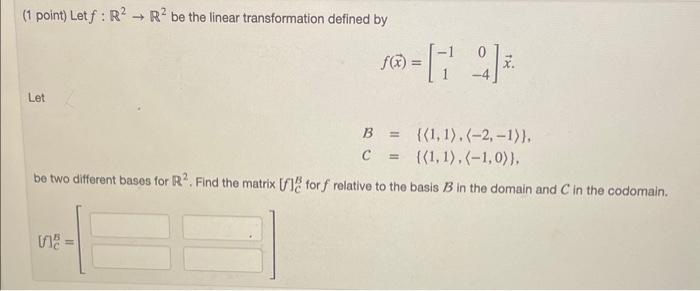
(1 point) Let \( f: \mathbb{R}^{2} \rightarrow \mathbb{R}^{2} \) be the linear transformation defined by \[ f(\vec{x})=\left[\begin{array}{cc} -1 & 0 \\ 1 & -4 \end{array}\right] \vec{x} . \] Let \[ \begin{array}{l} B=\{\langle 1,1\rangle,\langle-2,-1\rangle\}, \\ C=\{\langle 1,1\rangle,\langle-1,0\rangle\} . \end{array} \] be two different bases for \( \mathbb{R}^{2} \). Find the matrix \( [f]_{C}^{B} \) for \( f \) relative to the basis \( B \) in the domain and \( C \) in the codomain. \[ [f]_{C}^{E}=[ \]