(Solved): 1) Find the inverse of the given functions: (a) \( f(x)=\frac{x}{2}+3 \) (b) \( f(x)=\sqrt{x+3} \), ...

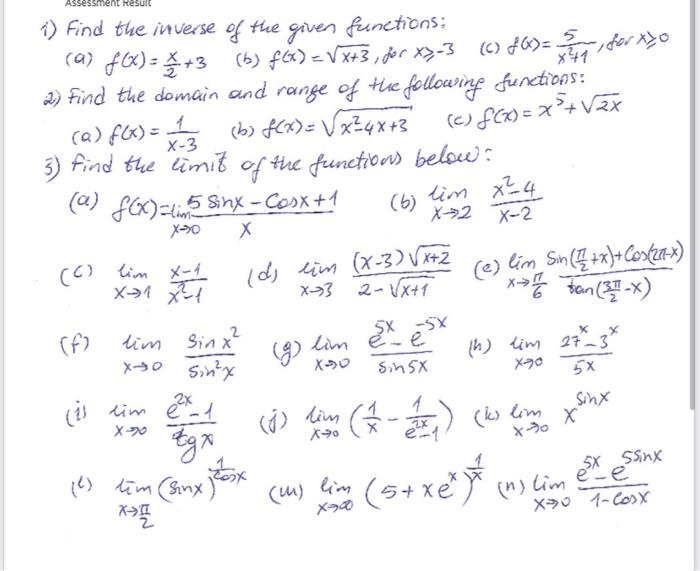
1) Find the inverse of the given functions: (a) \( f(x)=\frac{x}{2}+3 \) (b) \( f(x)=\sqrt{x+3} \), for \( x \geqslant-3 \) (c) \( f(x)=\frac{5}{x^{2}+1} \), for \( x \geqslant 0 \) 2) Find the domain and range of the following functions: (a) \( f(x)=\frac{1}{x-3} \) (b) \( f(x)=\sqrt{x^{2}-4 x+3} \) (c) \( f(x)=x^{5}+\sqrt{2 x} \) 5) Find the eimit of the functions below: (a) \( f(x)=\lim _{x \rightarrow 0} \frac{5 \sin x-\cos x+1}{x} \) (b) \( \lim _{x \rightarrow 2} \frac{x^{2}-4}{x-2} \) (c) \( \lim _{x \rightarrow 1} \frac{x-1}{x^{2}-1} \) (d) \( \lim _{x \rightarrow 3} \frac{(x-3) \sqrt{x+2}}{2-\sqrt{x+1}} \) (e) \( \lim _{x \rightarrow \frac{\pi}{6}} \frac{\sin \left(\frac{\pi}{2}+x\right)+\cos (2 \pi-x)}{\tan \left(\frac{3 \pi}{2}-x\right)} \) (f) \( \lim _{x \rightarrow 0} \frac{\sin x^{2}}{\sin ^{2} x} \) (g) \( \lim _{x \rightarrow 0} \frac{e^{5 x}-e^{-5 x}}{\sin 5 x} \) (h) \( \lim _{x \rightarrow 0} \frac{27^{x}-3^{x}}{5 x} \) (i) \( \lim _{x \rightarrow 0} \frac{e^{2 x}-1}{\operatorname{tg} x} \) (j) \( \lim _{x \rightarrow 0}\left(\frac{1}{x}-\frac{1}{e^{2 x}-1}\right) \) (k) \( \lim _{x \rightarrow 0} x^{\sin x} \) (l) \( \lim _{x \rightarrow \frac{\pi}{2}}(\sin x)^{\frac{1}{\cos x}} \) (m) \( \lim _{x \rightarrow \infty}\left(5+x e^{x}\right)^{\frac{1}{x}} \) (n) \( \lim _{x \rightarrow 0} \frac{e^{5 x}-e^{5 \sin x}}{1-\cos x} \)