(Solved): 1. Fill in the blank: Every bipartite graph has a chromatic number of 2. For each of the graphs be ...

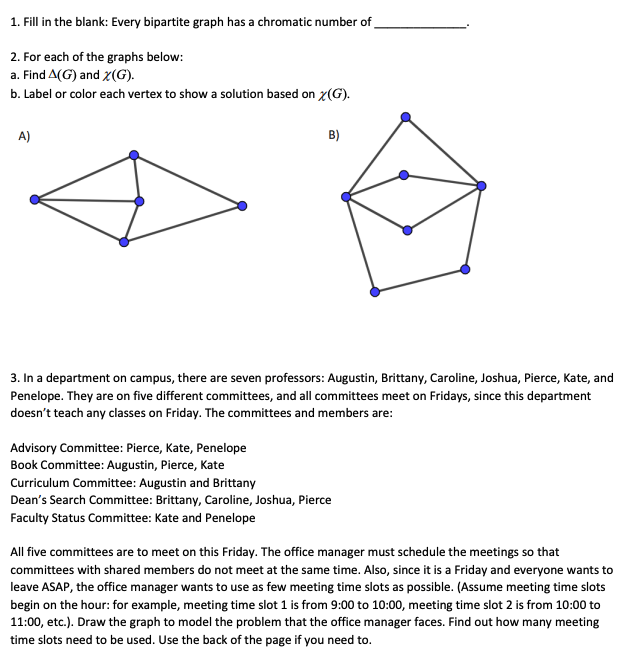
1. Fill in the blank: Every bipartite graph has a chromatic number of 2. For each of the graphs below: a. Find \( \Delta(G) \) and \( \chi(G) \). b. Label or color each vertex to show a solution based on \( \chi(G) \). 3. In a department on campus, there are seven professors: Augustin, Brittany, Caroline, Joshua, Pierce, Kate, and Penelope. They are on five different committees, and all committees meet on Fridays, since this department doesn't teach any classes on Friday. The committees and members are: Advisory Committee: Pierce, Kate, Penelope Book Committee: Augustin, Pierce, Kate Curriculum Committee: Augustin and Brittany Dean's Search Committee: Brittany, Caroline, Joshua, Pierce Faculty Status Committee: Kate and Penelope All five committees are to meet on this Friday. The office manager must schedule the meetings so that committees with shared members do not meet at the same time. Also, since it is a Friday and everyone wants to leave ASAP, the office manager wants to use as few meeting time slots as possible. (Assume meeting time slots begin on the hour: for example, meeting time slot 1 is from 9:00 to 10:00, meeting time slot 2 is from 10:00 to 11:00, etc.). Draw the graph to model the problem that the office manager faces. Find out how many meeting time slots need to be used. Use the back of the page if you need to.