Home /
Expert Answers /
Physics /
1-a-cylinder-of-density-pc-length-l-and-cross-section-area-a-floats-in-between-the-interface-of-t-pa114
(Solved): 1. A cylinder of density Pc, length l, and cross-section area A floats in between the interface of t ...
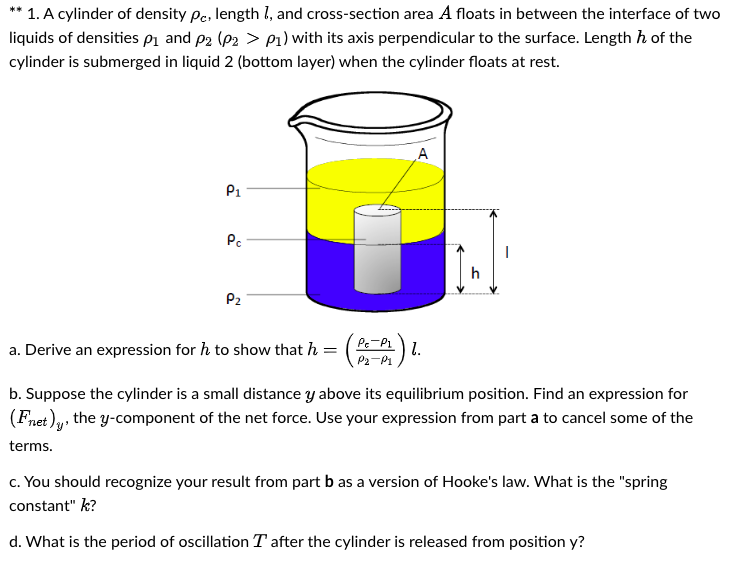
1. A cylinder of density Pc, length l, and cross-section area A floats in between the interface of two liquids of densities P1 and P2 (P2>P1) with its axis perpendicular to the surface. Length ℎ of the cylinder is submerged in liquid 2 (bottom layer) when the cylinder floats at rest.
a. Derive an expression for ℎ to show that ℎ=(Pc−P1/P2−P1)L.
b. Suppose the cylinder is a small distance Y above its equilibrium position. Find an expression for (Fnet)Y, the Y-component of the net force. Use your expression from part a to cancel some of the terms.
c. You should recognize your result from part b as a version of Hooke's law. What is the "spring constant" k?
d. What is the period of oscillation T after the cylinder is released from position y?
* 1. A cylinder of density , length , and cross-section area floats in between the interface of two liquids of densities and with its axis perpendicular to the surface. Length of the cylinder is submerged in liquid 2 (bottom layer) when the cylinder floats at rest. a. Derive an expression for to show that . b. Suppose the cylinder is a small distance above its equilibrium position. Find an expression for , the -component of the net force. Use your expression from part a to cancel some of the terms. c. You should recognize your result from part b as a version of Hooke's law. What is the "spring constant" ? d. What is the period of oscillation after the cylinder is released from position ?